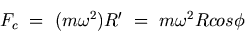Previous Contents Next College Physics: Gravitation G.5 Factors affecting Acceleration due to Gravity G.5.1 Altitude Consider a body at a height h above the surface of the Earth. Let the radius of the Earth be R and its mass be M. The body is at a distance (R + h) from the center of the Earth. If gh is the value of acceleration due to gravity at that point, then (G.5) Equation (G.5) when divided by the value of acceleration due to gravity at the Earth's surface (g = GM/R 2) gives (G.6) If h is much smaller than R, then equation (G.6) can be rewritten using binomial expansion as (G.7) Thus the value of gravitational acceleration decreases as one moves away from the surface of the Earth. Click here for Solved Example G.5: Acceleration due to Gravity at a Height G.5.2 Depth Below the Earth's Surface Consider a body taken to a depth h inside the Earth's surface. The body will be attracted by the mass of the Earth which is enclosed in a sphere of radius (R - h). If the mass of this portion is denoted by M', the acceleration due to gravity at the point by g'h and the density of the Earth by , then (G.8) Now, acceleration due to gravity at the surface can be written as (G.9) Dividing equation (G.8) by equation (G.9), we obtain (G.10) The value of gravitational acceleration decreases if one moves towards the center of the Earth, e.g., in mines. Thus, the value of gravity is maximum at the Earth's surface and decreases with increase in height as well as with depth. Click here for Solved Example G.6: Acceleration due to Gravity at a Depth G.5.3 Rotation of the Earth Assume the Earth to be a sphere of radius R and mass M rotating on its axis with an angular velocity w. Consider a particle of mass m at P such that OP makes an angle of ø with OE (Figure G.1). Here ø is the latitude of the particle. The particle is moving in a circle of radius R' = R cos ø. Figure G.1 Effect of the Earth's Rotation on gravitational acceleration. The net force pulling the particle towards the center of the Earth is (G.11) where FN is the total force acting on the particle, FG is the force due to gravity and Fc is the centrifugal force. The centrifugal force is given by (G.12) On substituting the appropriate expressions in equation (G.11), we obtain (G.13) If g' represents the gravitational acceleration, then (G.14) (G.15) Thus the observed value of gravitational acceleration is minimum at the equator and maximum at the poles.   Previous Contents Next

 

English
Français
Português
Español
Deutsch
日本語
हिन्दी