Solution:

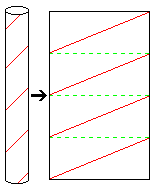
To make visualization easy, it is convenient to conceptually open out the bark of the tree trunk and flatten it. The cylindrical surface will then be a rectangle (as illustrated in the figure alongside for 4 twists of the creeper).

It may be noted that:
Width of the rectangle = Circumference of the cylinder = 48 inches.

Height of the rectangle = Vertical distance on the cylinder = 90 inches (in one twist).

Using the Pythagorean Theorem for a right-angled triangle,

Length of the hypotenuse = (48² + 90²) ^1/2 = 102 inches.

Now, the number of twists the creeper makes around the tree trunk is 5 (= 450 / 90).

If the length of the creeper (as given by the hypotenuse) is 102 inches in one twist, then the total length of the creeper in 5 twists is 510 inches.

Food for thought:

Is the total length of the creeper in the above calculation the actual length or the minimum length?