|
Heat Transfer Problem :
Heat conduction from a sphere to a stagnant fluid
Problem.
A heated sphere of diameter D is placed in a large amount of stagnant fluid. Consider the heat conduction in the fluid surrounding the sphere in the absence of convection. The thermal conductivity k of the fluid may be considered constant. The temperature at the sphere surface is TR and the temperature far away from the sphere is Ta.
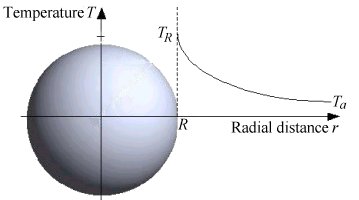Figure. Heated sphere in a large amount of stagnant fluid.
a) Establish an expression for the temperature T in the surrounding fluid as a function of r, the distance from the center of the sphere.
b) If h is the heat transfer coefficient, then show that the Nusselt number (dimensionless heat transfer coefficient) is given by
Hint: Equate the heat flux at the sphere surface to the heat flux given by Newton's law of cooling.
a)
From a heat balance over a thin spherical shell in the surrounding fluid,
 |
(1) |
where S is the rate of generation of heat per unit volume. In this case, S = 0 in the fluid.
Since the thermal conductivity k for the fluid is constant, on substituting Fourier's law ( ) we get
 |
(2) |
On integrating,
 |
(3) |
The integration constants are determined using the boundary conditions:
 |
(4) |
 |
(5) |
where R is the radius of the sphere.
On substituting the integration constants, the temperature profile is
 |
(6) |
b)
Using Fourier's law and differentiating the temperature profile, the heat flux is
 |
(7) |
Equating the heat flux at the sphere surface (r = R) to the heat flux as per Newton's law of cooling, we get
 |
(8) |
The Nusselt number (which is the dimensionless heat transfer coefficient) is
 |
(9) |
where D is the diameter of the sphere.
Note:
- This is a well-known result that is worth remembering. It provides the limiting value of the Nusselt number for heat transfer from a sphere in the presence of convection at low Reynolds and Grashof numbers.
-
The Nusselt number Nu must not be confused with the Biot number Bi. Though the two dimensionless groups are similar-looking, they differ as given below.
 |
(10) |
 |
(11) |
Syvum in other languages: French, Spanish, Portuguese
|
