Statement (1) specifies that x < −1, which implies −x > 1, −1 < (1/x) < 0 and −x > (1/x).
Thus, statement (1) ALONE is sufficient.
Statement (2) specifies that |x| > 0, which implies x > 0 or x < 0.
If x > 0, then −x is negative, (1/x) is positive and −x < (1/x).
If x < 0, then −x is positive, (1/x) is negative and −x > (1/x).
Thus, statement (2) ALONE is not sufficient.

Statement (1) specifies that the price of both types of olives is the same, but does not give a numerical value for the price. Thus, statement (1) ALONE is not sufficient.
Statement (2) gives the following equation:
9b + 4g = 26,
where bis the price of a bottle of black olives and g is the price of a bottle of green olives.
Note that when this equation is multiplied by 10 throughout, the price of 90 bottles of black olives and 40 bottles of green olives is obtained. Thus, statement (2) ALONE is sufficient.

Statement (1) only tells us that Δ ABC is isosceles which is not enough to find the area of the triangle. Thus, statement (1) ALONE is not sufficient.
Statement (2) specifies the length of the base, but does not specify the altitude (or height) of the triangle. Thus, statement (2) ALONE is not sufficient.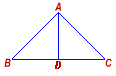
In an isosceles triangle, the altitude from the vertex angle bisects the base (as shown in the figure alongside). So, BD = ½ BC. Knowing ABand BD, the altitude AD can be found by the Pythagorean theorem. Since area of triangle = ½ base x altitude, the area can be determined as ½ BC x AD. Thus, BOTH statements TOGETHER are sufficient.

The quadrant in which the point lies can be determined if the sign (+ or −) of both xand y are known.
Statement (1) gives two linear equations that may be solved to determine the values of the two unknowns xand y.Thus, statement (1) ALONE is sufficient.
Statement (2) specifies the sign of both xand y to be negative. So, the point is in quadrant III. Thus, statement (2) ALONE is sufficient.
Therefore, EACH statement ALONE is sufficient.

Statement (1) specifies the price of each shirt, but not the number of shirts sold. Thus, statement (1) ALONE is not sufficient.
Statement (2) specifies the ratio of shirts to trousers, but nothing about their numbers. Thus, statement (2) ALONE is not sufficient.
The money collected from shirt sales can be found on multiplying the price per shirt by the number of shirts. Since the number of shirts sold cannot be obtained, statements (1) and (2) TOGETHER are NOT sufficient.

To find the perimeter of square, its side must be known because
Perimeter of square = 4 × Side.
Statement (1) specifies the side. Thus, statement (1) ALONE is not sufficient.
Statement (2) specifies the diagonal. The side of the square may be found using the Pythagorean theorem and then the perimeter calculated as follows:
KL² + LM² = (√32)²
Since KL = LM, 2LM² = 32 or LM = √16 = 4 inches.
Perimeter of square = 4 × 4 inches = 16 inches.
Thus, statement (2) ALONE is sufficient.

Distance of point (m, n) from origin = m² + n²
Distance of point (p, q) from origin = p² + q²
Difference in distances = (m² + n²) −(p² + q²)
= (m + n)² − 2mn −(p + q)² + 2pq
= (m + n)² − (p + q)² − 2(mn − pq)
Statement (1) specifies (mn − pq), whereas statement (2) specifies (p + q). Since there is no information available on (m + n), statements (1) and (2)
TOGETHER are NOT sufficient.

Let sand b be the number of small and big boxes, respectively.
Then, total number of discs t = 20s = 50b.
Statement (1) specifies t = 6000, which gives b = 6000/50.Thus, statement (1) ALONE is sufficient.
Statement (2) specifies b/s = 2/5; however, this information is already known from 20s = 50b. Thus, statement (2) ALONE is not sufficient.

Let Tand G denote the values of televisions and goods, respectively.
Then, T₂₀₀₀ = 0.234 G₂₀₀₀.
Required percent change = (1 − T₂₀₀₄/T₂₀₀₀) x 100%
Statement (1) gives T₂₀₀₄ = 0.178 G₂₀₀₄. Without knowing the values of G₂₀₀₀and G₂₀₀₄, the required percent change cannot be determined. Thus, statement (1) ALONE is not sufficient.
Statement (2) specifies G₂₀₀₀/G₂₀₀₄= 9/10. Without knowing the value of T₂₀₀₄, the required percent change cannot be found. Thus, statement (2) ALONE is not sufficient.
On combining both statements, the required percent change = [1 − (0.178 G₂₀₀₄)/(0.234 G₂₀₀₀)] x 100% = [1 − (0.178 x 10)/(0.234 x 9)] x 100%. Thus, BOTH statements TOGETHER are sufficient.

Now, d = 3/(4y) + 4/(3y) = 9/(12y) + 16/(12y) = 25/(12y).
n/d = x/y × 12y/25 = 12x/25.
Statement (1) specifies the value of x, which allows determination of n/d. Thus, statement (1) ALONE is sufficient.
Statement (2) states that y = 2x. Without the explicit value of x, the quantity n/d cannot be determined. Thus, statement (2) ALONE is not sufficient.

From the figure, ∠ PSQ measures (180 − 2x)^o. Since the angles of the triangle PSQ must add to 180^o, ∠ PQS must measure x^o. Thus, PQS is an isosceles triangle with PS = QS.
In the figure, ∠ PQR measures 90^o. Since ∠ PQS measures x^o, ∠ RQS measures (90 − x)^o. Now, the angles of the triangle RQS must add to 180^o; so, ∠ QRS must measure (90 − x)^o. Thus, RQS is an isosceles triangle with RS = QS.
It may be therefore concluded that RS = QS = PS.
Statement (1) specifies ∠ QPS; however, the above analysis shows that the actual numerical value of the angle is not required to determineRS. Thus, statement (1) ALONE is not sufficient.
Statement (2) specifies PS, and RS = PS from the preceding analysis. Thus, statement (2) ALONE is sufficient.

Using the Pythagorean Theorem for right-angled triangle ABD gives
AB² = AD² + BD² ...(1)
Using the Pythagorean Theorem for right-angled triangle BCD gives
BC² = BD² + CD² ...(2)
Note that an angle inscribed in a semicircle is a right angle. Since AC is a diameter, ∠ ABC measures 90^o and ABC is a right-angled triangle.
Using the Pythagorean Theorem for right-angled triangle ABC gives
(AD + CD)² = AB² + BC² ...(3)
Expanding the left-hand side above, and substituting from equations (1) and (2) into the right-hand side gives
AD² + CD² + 2 AD CD =AD² + BD² + BD² + CD² ...(4)
Simplifying equation (4) gives
AD CD = BD² ...(5)
Statement (1) specifies AB. Since BD is given in the question statement, AD may be first calculated from equation (1) and then CD from equation (5). The diameter AC is simply the sum of ADand CD. Thus, statement (1) ALONE is sufficient.
Statement (2) specifies BC. Since BD is given in the question statement, CD may be first calculated from equation (1) and then AD from equation (5). The diameter AC is simply the sum of CDand AD. Thus, statement (2) ALONE is sufficient.
Therefore, EACH statement ALONE is sufficient.

Since the angles of a triangle add to 180^o, ∠ A + ∠ B + ∠ C = 180^o.
Statement (1) states that ∠ B = ∠ C. Since∠ B = 36^o, ∠ A may be calculated from∠ A = 180^o − 2 ∠ B. Thus, statement (1) ALONE is sufficient.
Statement (2) states that two sides of the triangle are equal in length,which implies the triangle is isosceles with ∠ B = ∠ C.As before, given ∠ B = 36^o, ∠ A may be calculated from ∠ A = 180^o − 2 ∠ B.Thus, statement (2) ALONE is sufficient.
Therefore, EACH statement ALONE is sufficient.

Let Mand W denote the number of men and women, respectively. Further, let subscripts V and N denote those who voted and those who did not vote, respectively. Then, M_V + W_V + M_N + W_N = 160000, and what is required is M_N + W_N.
Statement (1) states that W_N = 0.44 (W_N + W_V), which yields W_V = (0.56/0.44) W_N. Thus, statement (1) ALONE is not sufficient.
Statement (2) states that M_V = 0.63 (M_V + M_N), which yields M_V = (0.63/0.37) M_N. Thus, statement (2) ALONE is not sufficient.
On combining both statements, one obtains (1 + 0.63/0.37)M_N + (1 + 0.56/0.44)W_N = 160000. It is still not possible to evaluate (M_N + W_N) without knowing the value of at least one more variable. Thus, statements (1) and (2) TOGETHER are NOT sufficient.

Let D and H denote David's weight and Harry's weight, respectively. Then, it is given that D + H = 260.
Statement (1) states that H = 1.5 D, which may be used to eliminate H and solve for D. Thus, statement (1) ALONE is sufficient.
Statement (2) states that H = D + 52, which may be independently used to eliminate H and solve for D. Thus, statement (2) ALONE is sufficient.
Therefore, EACH statement ALONE is sufficient.